In an equilateral triangle with a height of
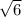 and one side measuring x, the length of side x is
and one side measuring x, the length of side x is
 in simplest radical form with a rational denominator.
in simplest radical form with a rational denominator.
In an equilateral triangle, the height (h) can be related to the side length (x) using the Pythagorean theorem. The formula is:
![\[ h^2 + \left((x)/(2)\right)^2 = x^2 \]](https://img.qammunity.org/2023/formulas/mathematics/high-school/tkjq9wbdt0unr8amp47z8ki9ged6d3ydhi.png)
Given that the height is
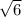 , substitute
, substitute
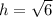 into the equation:
into the equation:
![\[ (√(6))^2 + \left((x)/(2)\right)^2 = x^2 \]\[ 6 + (x^2)/(4) = x^2 \]](https://img.qammunity.org/2023/formulas/mathematics/high-school/rac8v6ebtosyiztt88mlqivr70wcc1yk29.png)
Now, solve for x:
![\[ (3x^2)/(4) = 6 \]\[ x^2 = (24)/(3) \]\[ x^2 = 8 \]\[ x = √(8) \]\[ x = 2√(2) \]](https://img.qammunity.org/2023/formulas/mathematics/high-school/h0t7nkw3bkkymj58f3r7fer7wian3vspmz.png)
Therefore, the length of side x in simplest radical form with a rational denominator is
 .
.