Question

PLEASE HELP! A rock is thrown at 30 m/s horizontally off a cliff into the ocean below. The rock hits the water 2.0 seconds later.

How far did the rock travel horizontally? How high was the cliff? What was the rock's overall displacement? How fast was the rock traveling horizontally when it hit the water? How fast was the rock traveling vertically when it hit the water?
(pls explain step by step detail)

Answer 1

(Assumption: air resistance on the rock is negligible;
`g = 9.8\; {\rm m\cdot s^(-2)}`.)

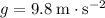The rock travelled
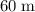`60\; {\rm m}` horizontally.

The cliff is approximately
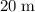`20\; {\rm m}` above the water.

The overall displacement of the rock is approximately
`63\; {\rm m}`.

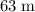Horizontal velocity of the rock right before it hit the water:
`30\; {\rm m\cdot s^(-1)}` (same as when the rock was launched.)

Vertical velocity of the rock right before it hit the water: approximately
`20\; {\rm m\cdot s^(-1)}`.

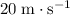Step-by-step explanation:

Assume that the air resistance on the rock is negligible. During the entire flight, the horizontal velocity of the rock will be constantly
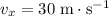`v_(x) = 30\; {\rm m\cdot s^(-1)}`. The vertical velocity of the rock will uniformly increase at a rate of
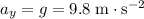`a_(y) = g = 9.8\; {\rm m\cdot s^(-2)}` (this rate is the vertical acceleration of the rock.)

Since the horizontal velocity of the rock is constant, multiply that velocity by time to find the horizontal displacement:

`\begin{aligned} x_(x) &= v_(x)\, t \\ &= 30\; {\rm m\cdot s^(-1)} * 2.0\; {\rm s} \\ &= 60\; {\rm m\cdot s^(-1)}\end{aligned}`.

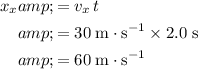Let
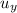`u_(y)` denote the initial vertical velocity of the rock. In this question,
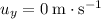`u_(y) = 0\; {\rm m\cdot s^(-1)}` since the stone was thrown horizontally.

While the vertical velocity of the rock isn't constant, vertical acceleration
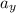`a_(y)` is constant. Hence, apply the SUVAT equation
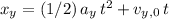`x_(y) = (1/2)\, a_(y)\, t^(2) + v_(y,0)\, t` to find the vertical displacement
`x_(y)`:

`\begin{aligned}x_(y) &= (1)/(2)\, a_(y)\, t^(2) + v_(y,0)\, t \\ &= (1)/(2)* 9.8\; {\rm m\cdot s^(-2)} * (2.0\; {\rm s})^(2) + 0\; {\rm m \cdot s^(-1)} * 2.0\; {\rm s} \\ &= (1)/(2)* 9.8* 2.0^(2) \; {\rm m} \\ &= 19.6\; {\rm m}\end{aligned}`.

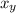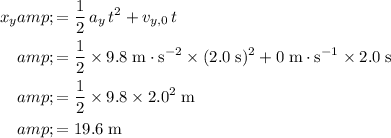The height of the cliff (relative to the water underneath) is equal to the vertical displacement of the rock:
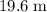`19.6\; {\rm m}` (approximately
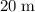`20\; {\rm m}` when rounded to two significant figures.)

The horizontal and vertical displacement of this rock are perpendicular to one another. These two displacements
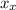`x_(x)` and
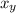`x_(y)` form the two sides of a right triangle, with the overall displacement
`x` as the other side (the hypotenuse.) The overall displacement of this rock can be found using the Pythagorean Theorem:

`\begin{aligned}x &= \sqrt{{x_(x)}^(2) + {x_(y)}^(2)} \\ &= \sqrt{(60\; {\rm m})^(2) + (19.6\; {\rm m})^(2)} \\ &\approx 63\; {\rm m}\end{aligned}`.

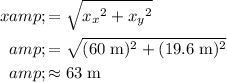Again, assume that air resistance on the rock is negligible. The horizontal velocity of the rock right before hitting the water will be the same as the initial value:
`30\; {\rm m\cdot s^(-1)}`.

Let
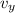`v_(y)` denote the vertical velocity of the rock right before hitting the water. Apply the SUVAT equation
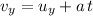`v_(y) = u_(y) + a\, t` to find
`v_(y)\!`:

`\begin{aligned} v_(y) &= u_(y) + a\, t \\ &= 0\; {\rm m\cdot s^(-1)} + 9.8\; {\rm m\cdot s^(-2)} * 2.0\; {\rm s} \\ &= 19.6\; {\rm m\cdot s^(-1)}\end{aligned}`.

Hence, right before hitting the water, the vertical velocity of this rock will be
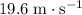`19.6\; {\rm m\cdot s^(-1)}` (rounded to
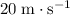`20\; {\rm m\cdot s^(-1)}`.)