Answer:
Line A: 4
Line B: -2
Explanation:
Note: gradient means slope, or m, in this context
To find the slope of a line, use the formula:
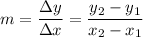 where
where
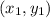 and
and
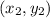 are points on the line
are points on the line
Line A has points (2, 2) and (3, 6). To find the slope of the line, simply plug these values into the formula for m :
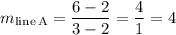
So, the slope, or gradient, of line A is 4.
Next, do the same thing for Line B.
Find two points on the line:
(0, 8) and (1, 6)
And plug these values into the formula:
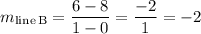
So, the slope, or gradient, of line B is -2.