Answer: All real numbers; (−∞ ,∞) ; infinite solutions
Explanation:
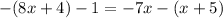
You want to distribute the (-) in (-(8x +4)) and (- (x + 5))
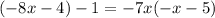
Combine like Terms on Both sides:
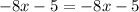
Add 5 to the right, and Add 8x to the left. (you want to cancel)
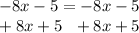
You are left with:
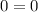
If you are end with 0=0, that means both equations are equal regardless of the variables. Therefore the answer is all real numbers; (−∞ ,∞) ; infinite solutions. You could check by substituting any values, and getting the same answers on both sides.