Answer:
Explanation:
We are given the position function:
s(t) = 183 - 16
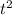
The velocity at t is the derivative of s(t), s'(t)
s'(t) = -32t
Given that the motion is in one direction, the speed would just be the magnitude of the velocity, or in this case the absolute value.
The acceleration at t is the second derivative of s(t), s''(t)
s''(t) = -32
The object will hit the ground when s(t) = 0
0 = 183 - 16
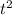
16
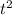 = 183
= 183
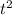 = 183/16
= 183/16
t =
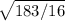
The objects velocity at the moment of impact is simply t we solved for earlier substituted into s'(t).
s'(sqrt(183/16)) = -32 * \sqrt{183/16}