Answers:
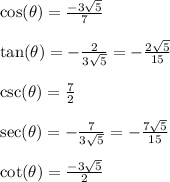
=================================================
Step-by-step explanation:
We're given that

Plug that into the pythagorean trig identity
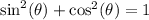 and solve for cosine to find that
and solve for cosine to find that

I skipped steps in solving so let me know if you need to see them.
Keep in mind that cosine is negative in quadrant 2
------------------
Once you've determined cosine, divide sine over cosine to get tangent
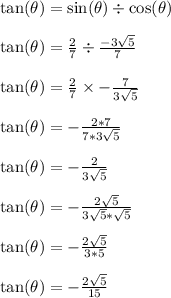
------------------
To determine cosecant, we apply the reciprocal to sine.
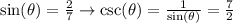
Similarly, secant is the reciprocal of cosine
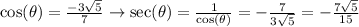
Depending on your teacher, rationalizing the denominator may be optional.
Lastly, cotangent is the reciprocal of tangent
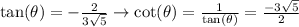
------------------
Side notes:
Sine and cosecant are the only things positive in Q2
Everything else (cosine, tangent, secant, cotangent) are negative in Q2.