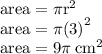Question

What is the shaded area???

Answer 1

Area of circle is 9π cm²

Explanation:

- If area of the area of the square is 36 cm², the length of one side of a square can be found;

`{ \rm{area \: of \: square = side * side}} \\ { \rm{36 = {x}^(2) }} \\ { \rm{x = √(36) }} \\ { \rm{x = 6 \: cm}}`

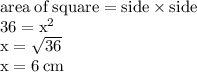
- If you make a clear observation from the figure, length of a square corresponds to the diameter of a circle;

`{ \rm{radius = (1)/(2) * length \: of \: square }} \\ \\ { \rm{r = (1)/(2) x}} \\ \\ { \rm{r = (1)/(2) * 6 }} \\ \\ { \rm{r = 3 \: cm}}`

- Therefore, let's find the area;

`{ \rm{area = \pi {r}^(2) }} \\ { \rm{area = \pi {(3)}^(2) }} \\ { \rm{area = 9\pi \: {cm}^(2) }}`

`{ \boxed{ \red{ \delta}}}{ \underline{ \mathfrak{ \: creed \: ...}}}`