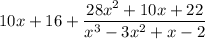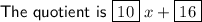Question

NEED HELP WITH THIS MATH QUESTION QUICK!

Answer 1

`\textsf{The quotient is $\boxed{10}\;x+\boxed{16}$}`

`\textsf{The remainder is $\boxed{28}\:x^2+\boxed{10}\:x+\boxed{22}$}`

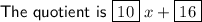
Explanation:

Definitions

Dividend: The polynomial which has to be divided.

Divisor: The expression by which the dividend is divided.

Quotient: The result of the division.

Remainder: The part left over.

Long Division Method of dividing polynomials

• Divide the first term of the dividend by the first term of the divisor and put that in the answer.
• Multiply the divisor by that answer, put that below the dividend and subtract to create a new polynomial.
• Repeat until no more division is possible.
• Write the solution as the quotient plus the remainder divided by the divisor.

Given:

`\textsf{Dividend}: \quad 10x^4-14x^3-10x^2+6x-10`

`\textsf{Divisor}: \quad x^3-3x^2+x-2`

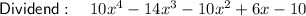
Therefore:

`\large \begin{array}{r}10x+16\phantom{)}\\x^3-3x^2+x-2{\overline{\smash{\big)}\,10x^4-14x^3-10x^2+6x-10\phantom{)}}}\\{-~\phantom{(}\underline{(10x^4-30x^3+10x^2-20x)\phantom{-b)}}\\16x^3-20x^2+26x-10\phantom{)}\\-~\phantom{()}\underline{(16x^3-48x^2+16x-32)\phantom{}}\\28x^2+10x+22\phantom{)}\\\end{array}`

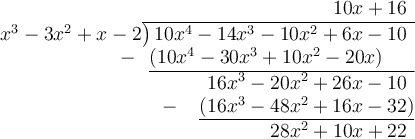
Solution:

`10x+16+(28x^2+10x+22)/(x^3-3x^2+x-2)`

`\textsf{The quotient is $\boxed{10}\;x+\boxed{16}$}`

`\textsf{The remainder is $\boxed{28}\:x^2+\boxed{10}\:x+\boxed{22}$}`