Answer:
Assume that the air resistance on the supplies is negligible, and that
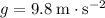 .
.
The plane need to drop the supplies when it is horizontally approximately
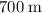 away from the hill.
away from the hill.
The supplies will hit the tree.
Step-by-step explanation:
Let
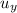 and
and
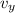 denote the initial and final vertical velocity of the supply;
denote the initial and final vertical velocity of the supply;
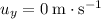 since the plane was flying horizontally.
since the plane was flying horizontally.
Let
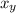 denote the vertical displacement of the supply;
denote the vertical displacement of the supply;
 .
.
Let
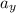 denote the vertical acceleration of the supply;
denote the vertical acceleration of the supply;
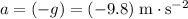 .
.
Make use of the SUVAT equation
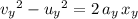 to find
to find
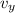 , the final vertical velocity of the supply:
, the final vertical velocity of the supply:
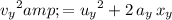 .
.
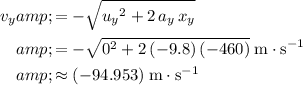 .
.
(Negative since the supply would be travelling downwards.)
Let
 denote time it takes for the supply to land on the hill after being dropped from the plane. Make use of the SUVAT equation
denote time it takes for the supply to land on the hill after being dropped from the plane. Make use of the SUVAT equation
 to find the value of
to find the value of
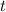 :
:
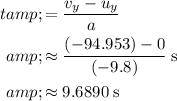 .
.
Apply unit conversion and ensure that
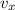 , the horizontal speed of the plane is in the standard unit
, the horizontal speed of the plane is in the standard unit
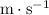 :
:
 .
.
Under the assumptions, the horizontal speed of the supply will be the same as that of the plane-
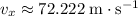 - until it lands.
- until it lands.
While in the air, the supply will travel a horizontal distance of:
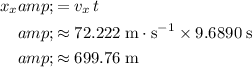 .
.
Hence, for the supply to land exactly at the top of the hill, the plane need to drop the supply while at a horizontal distance of approximately
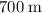 away from the hill.
away from the hill.
The horizontal distance between the trees and the location where the plane dropped the supply would be approximately
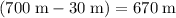 . The time required for the the supply to reach that horizontal position would be:
. The time required for the the supply to reach that horizontal position would be:
 .
.
Let
 denote the initial height of the supply (relative to the sea level.) In this question,
denote the initial height of the supply (relative to the sea level.) In this question,
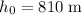 .
.
Let
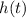 denote the height of the supply (relative to the sea level) after being dropped from the plane for time
denote the height of the supply (relative to the sea level) after being dropped from the plane for time
 .
.
The SUVAT equation
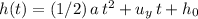 gives an expression for
gives an expression for
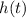 . Make use of this equation to find the height of the supply (relative to the sea level) when the supply reach the horizontal position of the trees at
. Make use of this equation to find the height of the supply (relative to the sea level) when the supply reach the horizontal position of the trees at
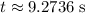 :
:
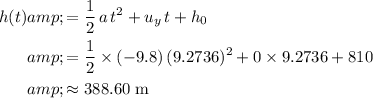 .
.
Note that the altitude of the top of the trees is
 relative to the sea level. Since
relative to the sea level. Since
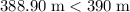 , the supplies will run into the trees.
, the supplies will run into the trees.