Answer:
10.97 seconds
Explanation:
You want to know when a rocket will hit the ground if its height is given by y = -16x² +170x +61, where x is seconds after launch.
Quadratic Formula
The formula for the solutions of a quadratic equation is ...
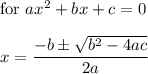
When we apply this to the equation y=0, we have ...
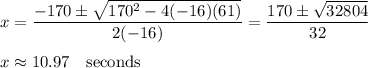
The rocket will hit the ground after about 10.97 seconds.
<95141404393>