Answer: Choice D
Step-by-step explanation
First, subtract Ax from both sides:
Ax - Ax + By = C - Ax
By = C - Ax
Now, divide B from both sides:
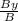 =
=
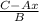
y =
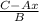
Finally, to get an equivalent answer for an answer choice, divide each term by B on the right side of the equation:
y =
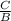 -
-
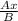
or
y = -
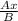 +
+
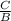
or
y =
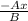 +
+
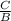
This answer is the same as answer choice D, and so D is the correct answer.