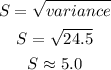1 Answer

Ask a Question

Volox · Answer 1 · 2023-01-07T20:27:04+0000

Answer:

Range = 15

Variance = 24.5Explanations

Standard deviation = 5.0

The formula for calculating the range is expressed as:

Range = Highest value - Lowest value

Range = 24 - 9

Range = 15

Calculate the variance

The formula for calculating the variance is expressed as:

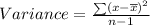
$Variance=\frac{\sum(x-\overline{x})^2}{n-1}$

Find the mean

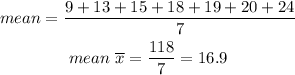
$\begin{gathered} mean=(9+13+15+18+19+20+24)/(7) \\ mean\text{ }\overline{x}=(118)/(7)=16.9 \end{gathered}$

Determine the variance

$\begin{gathered} S^2=((9-16.9)^2+(13-16.9)^2+(15-16.9)^2+(18-16.9)^2+(19-16.9)^2+(20-16.9)^2+(24-16.9)^2)/(7-1) \\ S^2=(146.9)/(6) \\ S^2\approx24.5 \end{gathered}$

Determine the standard deviation

$\begin{gathered} S=√(variance) \\ S=√(24.5) \\ S\approx5.0 \end{gathered}$

0 Comments

Please log in or register to add a comment.

Please log in or register to answer this question.

1 Answer

Answer:

0 Comments

Please log in or register to add a comment.

Other Questions