Given -
a = 2.5 cm
b = 3.6 cm
∠A = 43°
To Find -
Remaining angles and sides =?
Step-by-Step Explanation -
We will start by drawing the diagram:
Now, we know
By, sine rule:

Now, we know N
A + B + C = 180 c°°
43 + B + 79° = 180°°
B = 180° - 799 - 43°°
B = 58°
Now, Side b =
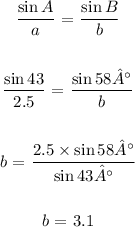
Final Answer -
∠B = 58B°
∠C = 797°
Side, b = 3.1 cm