Answer:
(2,3)
or (2,2) if the function does not need to be continuous.
Explanation:
For a set of points to be considered a function, it must be true that for every X value, you have only a single Y value solution.
In other words, looking through your points listed, you would remove any point where an X value appears twice with different Y values.
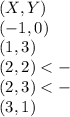
Notice the two points with arrows, both have 2 as their X value, but different Y values. Removing either one of them would make this a function. Although, by removing (2,3) the function has the potential to also be continuous, because having two of the same Y value for different X values would cause a piece-wise function which is generally non-continuous.