Question

MATH WIZARDS HELP PLEASE!!!!

Answer 1

Answer: 144 feet

=======================================================

Step-by-step explanation:

I'll use x in place of t

And use y in place of h(t)

The equation we have is y = 96x - 16x^2

This rearranges to y = -16x^2 + 96x

Compare this to y = ax^2+bx+c to find that

• a = -16
• b = 96
• c = 0

The x coordinate of the vertex is

h = -b/(2a)

h = -96/(2*(-16))

h = 3

Then plug this into the equation to find the value of y

y = -16x^2 + 96x

y = -16(3)^2 + 96(3)

y = 144

The y coordinate of the vertex is k = 144

The vertex is located at (h,k) = (3, 144) to represent the highest point of the parabola.

The max height of 144 feet occurs when the ball is in the air for 3 seconds.

Answer 2

What We Know

Equation: h(t) = 96t - 16t²

• This equation will form a parabola
• The zeros of the parabola will indicate the start time and height (0,0) and the end time and height (x, 0)
• The maximum point will be the maximum height the ball will reach
• The maximum point will be the halfway point between the two zeros

Step-by-Step

h(t) = 96t - 16
`t^(2)`

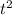
Find the zeros of the given equation:

Factor out the greatest common factor (GCF)

h(t) = 8t (12 - 2t)

The two factors are:

0 = 8t

0 = 12 - 2t

For both factors, solve for t

8t = 0

8t/8 = 0/8

t = 0

12 - 2t = 0

-2t = -12

t = 6

Now we have the x-values (t) of our zeros:

t = 0

(0, 0)

t = 6

(6, 0)

The ball will land on the ground at 6 seconds

Recall that the maximum point of the graph is halfway between the two zeros:

6 + 0 = 6

6 ÷ 2 = 3

The ball will reach its maximum height in 3 seconds

To find this height, substitute t for 3 in the given equation, and solve for h:

t = 3

h(3) = 96(3) - 16(3)²

= 288 - 16(9)

= 288 - 144

= 144

The maximum height the ball will reach is 144 feet

***Refer to the graph below to check your answer: