Answer:
48
Explanation:
Given:
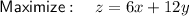
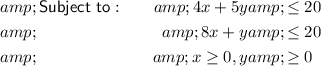
Graph the lines:
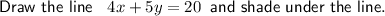
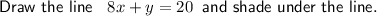

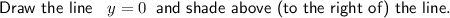
Therefore, the feasible region is bounded by the corner points:
- A = (0, 0)
- B = (0, 4)
- C = (⁵/₂, 0)
- D = (²⁰/₉, ²⁰/₉)
Determine the value of z at the corner points by substituting the x and y values of the points into the equation for z:
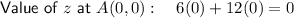
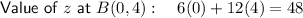

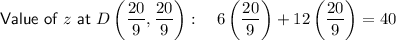
Hence, the maximum value of z is 48 at B(0, 4).