Answer: 2
===================================================
Step-by-step explanation:
The product of all diagonals, along with the two exterior sides, is equal to n which is the number of sides. This only applies if we're working with the unit circle. When I say "all diagonals", I'm referring to all diagonals from one fixed point. So the diagonals AC, AD, AE, all the way up to AJ. If we multiplied all of those diagonals together with sides AB and AK, then we'd get n = 11.
Now because this diagram only does half of what I mentioned, we have half as many products and we only get to
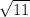 .
.
Comparing this to
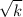 shows we have k = 11. The sum of the digits of k is 1+1 = 2.
shows we have k = 11. The sum of the digits of k is 1+1 = 2.
For more information, I recommend searching out "product of the diagonals of an inscribed polygon" without quotes.
The proof of this theorem involves complex numbers, stuff like 2+3i, so be sure to review that topic if necessary.