Answer:
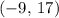 is in the second quadrant of a cartesian plane.
is in the second quadrant of a cartesian plane.
Explanation:
The horizontal and vertical axes divides the cartesian plane into four quadrants. The quadrants are numbered counterclockwise, starting from the top-right corner:
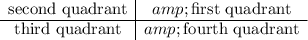 .
.
(The quadrants do not include the two axes. Points on either axes are not in any of the quadrant.)
A point in the form
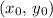 is on the right half of the plane (to the right of the vertical axis) if
is on the right half of the plane (to the right of the vertical axis) if
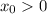 . This point is on the vertical axis through the middle of the plane if
. This point is on the vertical axis through the middle of the plane if
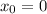 . Otherwise, if
. Otherwise, if
 , this point would be on the left half of the plane (to the left of the vertical axis.)
, this point would be on the left half of the plane (to the left of the vertical axis.)
The
 -coordinate of
-coordinate of
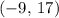 is
is
 .
.
Since the
 -coordinate of this point is below zero (
-coordinate of this point is below zero (
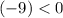 ), this point would be on the left-hand side of the origin to the left of the vertical axis.
), this point would be on the left-hand side of the origin to the left of the vertical axis.
Likewise, a point in the form
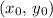 is above the horizontal axis if
is above the horizontal axis if
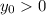 , on the horizontal axis if
, on the horizontal axis if
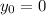 , and below the horizontal axis if
, and below the horizontal axis if
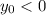 .
.
The
 -coordinate of the given point
-coordinate of the given point
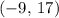 in this question is
in this question is
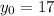 .
.
Since the
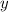 -coordinate of this point is greater than zero (
-coordinate of this point is greater than zero (
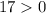 ), this point would be above the horizontal axis.
), this point would be above the horizontal axis.
Thus, the point
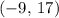 would be in the top-left quarter of the plane. The top-left quarter of the plane corresponds to the second quadrant, since this quadrant is the second quadrant after the first quadrant in the top-right corner. Hence,
would be in the top-left quarter of the plane. The top-left quarter of the plane corresponds to the second quadrant, since this quadrant is the second quadrant after the first quadrant in the top-right corner. Hence,
 would be in the second quadrant of the plane.
would be in the second quadrant of the plane.