Question

2. What is the slope of the line that passes through (3, 2) and (1, 8) equations?

Answer 1

slope = -3

Explanation:

ΔX = 1 – 3 = -2

ΔY = 8 – 2 = 6

Slope (m) =

Δ
`(Y)/(X) =(3)/(-1) = -3`

slope (m) = -3

Answer 2

Slope: -3

Equation: y = -3x + 11

Explanation:

Given points:

• (x₁, y₁) = (3, 2)
• (x₂, y₂) = (1, 8)

To find the slope of the line that passes through the given points, substitute the points into the slope formula:

`\implies \textsf{slope ($m$)}=(y_2-y_1)/(x_2-x_1)=(8-2)/(1-3)=(6)/(-2)=-3`

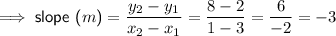
Therefore, the slope of the line that passes through (3, 2) and (1, 8) is -3.

To find the equation of the line that passes through the given points, substitute one of the points and the found slope into the point-slope form of a linear equation:

`\implies y-y_1=m(x-x_1)`

`\implies y-2=-3(x-3)`

`\implies y-2=-3x+9`

`\implies y-2+2=-3x+9+2`

`\implies y=-3x+11`

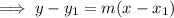
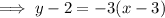
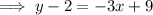
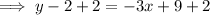
Therefore, the equation of the line that passes through (3, 2) and (1, 8) is:

`y=-3x+11`