Question

3x - 10 < 4(x - 2) Helpp please

Answer 1

`\boxed{\sf{x > -2}}`

Explanation:

To solve this, find the value of x to isolate it on one side of the equation.

3x-10<4(x-2)

Use the distributive property.

A(B+C)=AB+AC

4(x-2)

4*x=4x

4*2=8

Rewrite the equation.

4x-8

3x-10<4x-8

First, add by 10 from both sides.

`\longrightarrow \sf{3x-10+10 < 4x-8+10}`

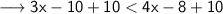
Solve.

3x<4x+2

Subtract by 4x from both sides.

`\sf{3x-4x < 4x+2-4x}`

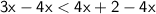
Solve.

-x<2

Then, you multiply by -1 from both sides.

(-x)(-1)>2(-1)

Solve.

Multiply these numbers from left to right.

2(-1)=-2

`\longrightarrow \boxed{\sf{x > -2}}`

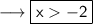
Therefore, the correct answer is x>-2.

I hope this helps, let me know if you have any questions.