Question

Which line is parallel to the line
shown below?

Answer 1

Answer: Choice D

4x - 3y = 15

====================================================

Step-by-step explanation:

The two points (-1,-1) and (2,3) are marked on the line

Let's find the slope of the line through those two points.

`(x_1,y_1) = (-1,-1) \text{ and } (x_2,y_2) = (2,3)\\\\m = (y_(2) - y_(1))/(x_(2) - x_(1))\\\\m = (3 - (-1))/(2 - (-1))\\\\m = (3 + 1)/(2 + 1)\\\\m = (4)/(3)\\\\`

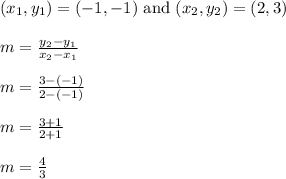
The slope is 4/3 meaning we go up 4 and to the right 3.

-------------

Parallel lines have equal slopes, but different y intercepts. We'll need to see which of the four answer choices have a slope of 4/3.

Solve the equation in choice A for y. The goal is to get it into y = mx+b form so we can determine the slope m.

`3x + 4y = -4\\\\4y = -3x-4\\\\y = -(3)/(4)x-(4)/(4)\\\\y = -(3)/(4)x-1`

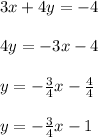
Equation A has a slope of -3/4 and not 4/3 like we want.

Therefore, this answer choice is crossed off the list.

Follow similar steps for choices B through D. I'll show the slopes of each so you can check your work.

• slope of equation B is 3/4
• slope of equation C is -4/3
• slope of equation D is 4/3

We have a match with equation D. Therefore, the equation 4x-3y = 15 is parallel to the given line shown in the graph.

You can use graphing tools like Desmos or GeoGebra to confirm the answer.