Answer: A. Both the slopes and y-intercepts are the same
Explanation:
We know that the y-intercepts are the same, because the linear function formula is written as y = mx + c, where c is the y-intercept. It is made quite clear in the graph that the line crosses the y-axis at y = 3, meaning the two intercepts are equal.
To assure the slopes are the same, we can use the slope formula:

We can now choose two coordinates to plug in here, I will use the ones shown on the graph, which are (1, 1) and (0, 3). We can then plug these into the formula:
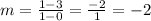
And we can see that g(x) has the same exact slope, making both identical.
Hope this helps, if you need anything else explained please let me know.