Answer:
1.42 m
Step-by-step explanation:
Let's first write down the data given
We have
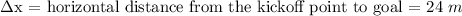
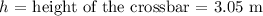
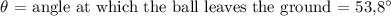
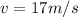
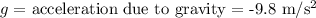 .
.
Note that we take g to -9.8 m/s² since gravity is acting in the opposite direction to the vertical movement of the ball. Thus the ball is slowing down with increasing height
The horizontal component of the velocity
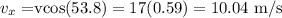
Time t, taken to traverse distance of 24m = Δx/vx = 24/10.04 = 2.39s
The vertical component of velocity
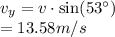
The vertical displacement is given by
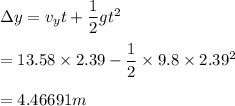
Since the height of the cross bar is 3.05m, the ball clears the crossbar by 4.46691 - 3.05 = 1.41691 ≈ 1.42 m