Question

Evaluate the given functions at the indicated value and simplify.

Answer 1

See Below

Explanation:

For each of these, you just replace x with what's in the parenthesis.

f(-2) = 4(-2)^2 - 2(-2) + 1

f(-2) = 16 + 4 + 1

f(-2) = 21

g(5) =
`√(4(5) - 16)`

g(5) =
`√(20-16)`

g(5) =
`√(4)`

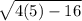
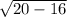
g(5) = 2

f(3r) = 4(3r)^2 - 2(3r) + 1

f(3r) = 4(9r^2) - 6r + 1

f(3r) = 36r^2 - 6r + 1

f(a+b) = 4(a+b)^2 - 2(a+b) +1

f(a+b) = 4 (a^2 + 2ab + b^2) - 2a - 2b + 1

f(a+b) = 4a^2 + 8ab + 4b^2 - 2a - 2b + 1