Answer:
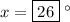
Explanation:
Intersecting Secants Theorem
If two secant segments are drawn to the circle from one exterior point, the measure of the angle formed by the two lines is half of the (positive) difference of the measures of the intercepted arcs.
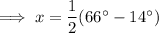
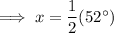
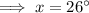
Circle Theorem vocabulary
- Secant: a straight line that intersects a circle at two points.
- Arc: the curve between two points on the circumference of a circle
- Intercepted arc: the curve between the two points where two chords or line segments (that meet at one point on the other side of the circle) intercept the circumference of a circle.