Question

2. Joel wants to have a halloween party. Basic expenses outside of food are $150. If it costs twelve dollars per

person, Joel wants to stay at or below $500. At most, many people can he invite? Write the equation and
answer the question. Assume x is the number of friends he can invite. HINT: the left side of the equation are
total expenses

Answer 1

x less than or equal to 29

Explanation:

This is an inequality I believe, therefore we start out with either a greater than less than or equal to sign, here he doesn't want to spend more than but he can spend $500 at most, so less than or equal to $500 you can also show it as
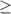`\geq`$500. So now that we have that lets go to the other side of the equation. If outright says he spends $150 at the start so now we have
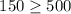`$150 \geq 500`, lets keep doing. is cost $12 per person, so lets use X to represent a person, now we have
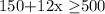`150 + $12x \geq $500`. Alright so now we have to see how many people or friends he can invite. So we get this by subtracting 150 from both sides. We do this because 150 is how much he has to spend, so we can subtract that from his spending limit, but we also remove this from the other side to keep the inequality balanced, now we have
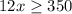`12x \geq 350`. We can now divide both sides by 12 because each person costs $12, so dividing that from the spending limit will give you the amount of people he can invite with the money. The number you would get would be 29.1 with a repeating 6, but you can't invite 29.1 people, you cant get a tenth of a person to come over, but he also can't get 30 because it would be above the limit, so he can only invite 29 people also shown by
`x \geq 29`