Answer:
r = 33 , s = 18
Explanation:
Rationalise the denominator by multiplying both numerator and denominator by the conjugate of the denominator.
The conjugate of 4 -
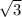 is 4 +
is 4 +
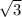 , then
, then
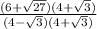 ← expand numerator/denominator using FOIL
← expand numerator/denominator using FOIL
=
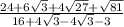
=

=
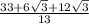
=
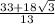
with r = 33 and s = 18