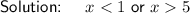Answer:
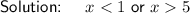

Explanation:
Given inequality:
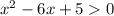
Factor the quadratic to find the x-intercepts
To factor a quadratic in the form
 , find two numbers that multiply to
, find two numbers that multiply to
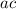 and sum to
and sum to
 :
:

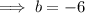
Therefore, the two numbers are: -1 and -5.
Rewrite
 as the sum of these two numbers and equal the quadratic to zero:
as the sum of these two numbers and equal the quadratic to zero:
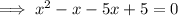
Factor the first two terms and the last two terms separately:

Factor out the common term (x - 1):
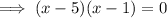
The x-intercepts are when the curve crosses the x-axis (when y=0).
Therefore, the x-intercepts are:
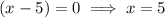

As the leading coefficient of the quadratic is positive, the parabola opens upwards. Therefore, to find the solution of the inequality, find the interval where the curve is positive (above the x-axis).
The curve is above the x-axis when x < 1 or x > 5.
Therefore, the solution to the quadratic inequality is: