Question

Find an equation of the line that satisfies the given conditions. Through (4, 9); parallel to the line passing through (5, 7) and (1, 3)

Answer 1

y = 1x + 5

Explanation:

First, lets calculate the equation of the line passing through (5, 7) and (1, 3) in slope-intercept form

The general equation of a line in slope-intercept form is
y = mx + b

where m = slope and b = y-intercept is rise over run =

`m = \frac {(y_(2) - y_(1))} {(x_(2) - x_(1))}`

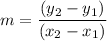
where (x₁, y₁) and (x₂, y₂) are any two points on the line

For the line passing through (5, 7) and (1, 3) , the slope

`m = (3 - 7)/(1 - 5)`

`m = (-4)/(-4)`

`m = 1`

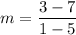
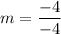
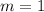
To compute the y-intercept, plug in any of the two points x and y values and solve for b

Take point 5, 7 ==> when x = 5, y must be 7

We get
7 = 1 x 5 + b
7 = 5 + b
2 = b (subtract 5 from both sides)
b = 2

So the equation of the line is
y = 1x + 2

A line parallel to another line will have the same slope and a different y-intercept

Therefore a parallel line will also have slope 1 and its equation will be of the form
y = 1x + b

To calculate this b, plug in the point (4, 9) into the equation and solve for b
9 = 1 x 4 + b
9 = 4 + b
b = 5

So the equation is
y = 1x + 5

Graph attached shows the real picture