Answer: a
Explanation:
First term
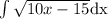
We can use the substitution u=10x-15, meaning dx = 1/10 du.
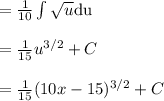
Second term

We can use the substitution u=10x-15, meaning dx = 1/10 du.
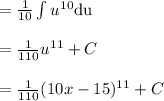
Third term
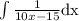
We can use the substitution u=10x-15, meaning dx = 1/10 du.
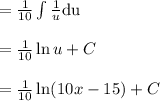
Adding these results, we get the answer to be a.