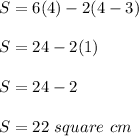Answer:
Explanation:
The area of a triangle is equal to half the product of the base by the height drawn to that base
Hence,
1)

2)
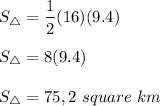
3)
The area of a trapezoid is equal to the product of the half sum of the trapezoid bases by the height
Hence,
1 ft=12 in
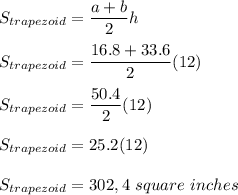
4)
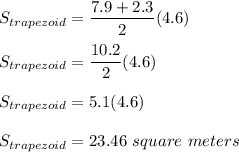
5)
The area of a rectangle is equal to the length times the width of its sides
Hence,
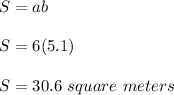
6)