Answer:
$3.90
Explanation:
Expected Value formula

where:
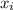 is an outcome.
is an outcome.
 is the probability of the outcome.
is the probability of the outcome.
Given table:

Substitute the given values into the Expected Value formula:

Therefore, the expected value is $3.90.
So, on average, you would expect to receive $3.90 in winnings per game.