Answer:
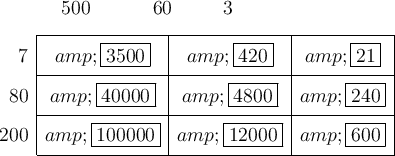
Explanation:
For each box, multiply the number of the row by the number of the column:
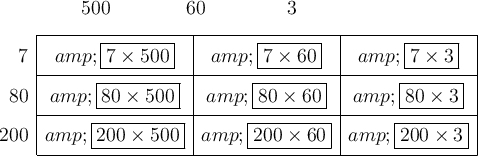
When multiplying numbers ending in zeros (multiples of 10, 100, etc.), first multiply the numbers as if they didn't have zeros, then add all the zeros to the final answer.
For example:
200 × 500 has a total of 4 zeros.
Multiply 2 × 5 = 10
Then add 4 zeros: 10 0000
Therefore, 200 × 500 = 100000
Final Solution
