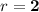Answer:
r = 2
Explanation:
To solve this problem, we have to use the following formula for slope:
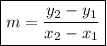 ,
,
where
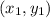 and
and
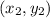 are the coordinates of two points.
are the coordinates of two points.
We are given the coordinates
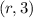 and
and
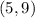 , and told that
, and told that
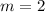 . To find the value of r, we have to substitute the given values into the formula and then solve for r :
. To find the value of r, we have to substitute the given values into the formula and then solve for r :
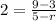
⇒
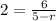
⇒
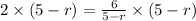 [Multiplying both sides by (5 - r)
[Multiplying both sides by (5 - r)
⇒
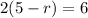
⇒
 [Dividing both sides by 2]
[Dividing both sides by 2]
⇒
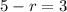
⇒
 [Subtracting 5 from both sides]
[Subtracting 5 from both sides]
⇒

⇒
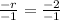 [Dividing both sides by -1]
[Dividing both sides by -1]
⇒