Question

Nb 3 all parts pls pls very important pls and thank you!!

Answer 1

Explanation:

(a)

First:

Get AE using Pythagoras

`{ae}^(2) = {6}^(2) + {8}^(2)`

`{ae}^(2) = 100`

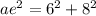
AE =10

Connect OM to form Traingle AOM.

AM=5cm (AE is 10 and AM=ME meaning that they are divided into two equal parts)

AM^2=OM^2+AO^2

5^2=OM^2+4^2

OM^2=5^2-4^2

OM=3

(B) OM is parallel to BE because of midpoint theorem

The Theorem states that The line segment in a triangle joining the midpoint of two sides of the triangle is said to be parallel to its third side and is also half of the length of the third side.

(C) AOM is a right angled triangle at O because angle AOM is 90°. Because OM is a perpendicular Line that bisects the chord of AB