Question

On a day when the wind is blowing toward the south at 3 m/s, a runner jogs west at 4 m/s. What is the speed of the

air relative to the runner?

Answer 1

`{ \qquad\qquad\huge\underline{{\sf Answer}}}`

Here we go ~

• 
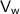  `{\sf {V}_(w) }`= velocity of wind = 3 m/s (south)

• 
  `{\sf {V}_(r) }`= velocity of runner = 4 m/s (west)

So,

• 
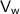  `{\sf {V}_(w) }` = - 3
  `{ \sf \hat{j}}`

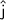
[ taking unit vector along north be
`{ \sf \hat{j}}` ]

• 
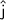  `{\sf {V}_(r) }` = - 4
  `{ \sf \hat{i}}`

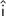
[ taking unit vector along west be
`{ \sf \hat{i}}` ]

`\qquad \dashrightarrow\sf \: V_(wr)= V_(w) - V_(r )`

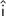[ where
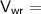`{ \sf V_(wr)= }` Relative velocity of wind with respect to runner ]

`\qquad \dashrightarrow\sf \: V_(wr)= - 3 \hat{ j} - ( - 4 \hat{i})`

`\qquad \dashrightarrow\sf \: V_(wr)= - 3 \hat{ j} + 4 \hat{i}`

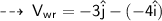
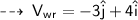
That is : 3 m/s towards south and 4 m/s towards east.

So, it makes an angle of 37° with south, and 53° with East.

[ The resultant is depicted by yellow arrow in attachment ]

Magnitude :

`\qquad \dashrightarrow\sf \: |V_(wr) | = \sqrt{3 {}^(2) + 4 {}^(2) }`

`\qquad \dashrightarrow\sf \: |V_(wr) | = √(9 + 16 )`

`\qquad \dashrightarrow\sf \: |V_(wr) | = √(25 )`

`\qquad \dashrightarrow\sf \: |V_(wr) | = 5 \: \: m/s`

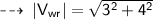
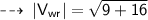
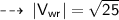
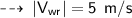
Direction :

`\dashrightarrow \sf \tan( \alpha) = (b\: \sin \theta)/(b + a \sin( \theta) )`

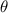`{\sf \theta }` = angle between the two vectors ( along east and south) i.e 90°

• b = 4 units

`\dashrightarrow \sf \tan( \alpha) = (4\: \sin (90 \degree))/(3+ 4 \cos( 90 \degree) )`

`\dashrightarrow \sf \tan( \alpha) = (4 * 1)/(3+( 4 * 0))`

`\dashrightarrow \sf \tan( \alpha) = (4)/(3)`

`\dashrightarrow \sf \alpha = \tan {}^( - 1) \bigg( (4)/(3 ) \bigg)`

`\dashrightarrow \sf \alpha = 53 \degree`

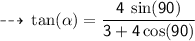
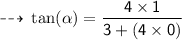
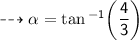
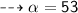
So, it's direction is 53° east from south ~