Question

Pls answer I report if wrong

Answer 1

Answer: Choice A

==========================================================

Step-by-step explanation:

Point L is at the location (-4, 2)

Translate 3 units to the left to arrive at (-7,2)

Then translate 4 units up to get (-7,6) which leads to choice A

---------------

The rule of "translate 3 units left, 4 units up" can be condensed into the notation of
`(\text{x},\text{y})\to(\text{x}-3,\text{y}+4)`

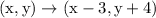
Let's apply this rule to point M(1,2)

`(\text{x},\text{y})\to(\text{x}-3,\text{y}+4)\\\\(1,2)\to(1-3,2+4)\\\\(1,2)\to(-2,6)\\\\`

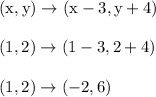
This further confirms what choice A is saying since it mentions M'(-2,6)

I'll let you check the other points in choice A.