Question

If √3 = 1.732, then √[(√3-1)/(√3+1)] is equal to​

Answer 1

Explanation:

Given Question :-

`\sf \: √(3) = 1.732, \: then \: \sqrt{( √(3) - 1)/( √(3) + 1) }`

`\red{\large\underline{\sf{Solution-}}}`

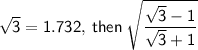
Given expression is

`\rm :\longmapsto\: \sqrt{( √(3) - 1)/( √(3) + 1) }`

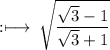
On rationalizing the denominator, we get

`\rm \:  =  \: \sqrt{( √(3) - 1)/( √(3) + 1) * ( √(3) - 1)/( √(3) - 1) }`

`\rm \:  =  \: \sqrt{\frac{ {( √(3) - 1)}^(2) }{( √(3) + 1)( √(3) - 1)} }`

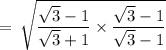
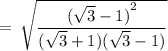
We know,

`\boxed{ \tt \: (x - y)(x + y) = {x}^(2) - {y}^(2) \: }`

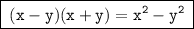
So, using this, we get

`\rm \:  =  \: \frac{ √(3) - 1}{ \sqrt{ {( √(3))}^(2) - {(1)}^(2) } }`

`\rm \:  =  \: ( √(3) - 1)/( √( 3 - 1) )`

`\rm \:  =  \: ( √(3) - 1)/( √(2) )`

`\rm \:  =  \: ( √(3) - 1)/( √(2) ) * ( √(2) )/( √(2) )`

`\rm \:  =  \: ((1.732 - 1) √(2) )/(2)`

`\rm \:  =  \: (0.732 * √(2) )/(2)`

`\rm \:  =  \: 0.366 √(2)`

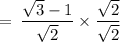
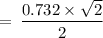
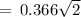
Hence,

`\rm :\longmapsto\: \boxed{ \rm{ \: \: \: \sqrt{( √(3) - 1)/( √(3) + 1) } = 0.366 √(2) \: \: \: }}`

▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬▬

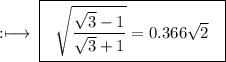
More Identities to know :-

(a + b)² = a² + 2ab + b²

(a - b)² = a² - 2ab + b²

a² - b² = (a + b)(a - b)

(a + b)² = (a - b)² + 4ab

(a - b)² = (a + b)² - 4ab

(a + b)² + (a - b)² = 2(a² + b²)

(a + b)³ = a³ + b³ + 3ab(a + b)

(a - b)³ = a³ - b³ - 3ab(a - b)