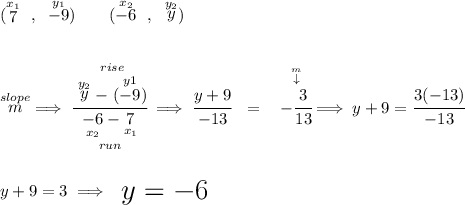Question

Given T(1,5), U(-2,-8), V(7,-9), and W(-6, y). Find y such that
TU VW

Answer 1

keeping in mind that perpendicular lines have negative reciprocal slopes, let's check for the slope of TU

`(\stackrel{x_1}{1}~,~\stackrel{y_1}{5})\qquad (\stackrel{x_2}{-2}~,~\stackrel{y_2}{-8}) ~\hfill \stackrel{slope}{m}\implies \cfrac{\stackrel{rise} {\stackrel{y_2}{-8}-\stackrel{y1}{5}}}{\underset{run} {\underset{x_2}{-2}-\underset{x_1}{1}}} \implies \cfrac{-13}{-3}\implies \cfrac{13}{3}`

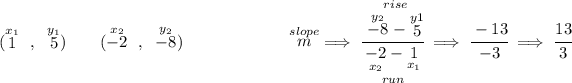
hmmmm, well, if that's so, then the slope of VW will be the negative reciprocal of that only if both are really perpendicular, so

`\stackrel{~\hspace{5em}\textit{perpendicular lines have \underline{negative reciprocal} slopes}~\hspace{5em}} {\stackrel{slope}{\cfrac{13}{3}} ~\hfill \stackrel{reciprocal}{\cfrac{3}{13}} ~\hfill \stackrel{negative~reciprocal}{-\cfrac{3}{13}}}`

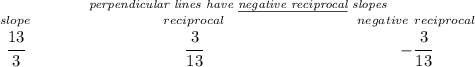
since now we know the slope if VW, let's use it

`(\stackrel{x_1}{7}~,~\stackrel{y_1}{-9})\qquad (\stackrel{x_2}{-6}~,~\stackrel{y_2}{y}) \\\\\\ \stackrel{slope}{m}\implies \cfrac{\stackrel{rise} {\stackrel{y_2}{y}-\stackrel{y1}{(-9)}}}{\underset{run} {\underset{x_2}{-6}-\underset{x_1}{7}}} \implies \cfrac{y +9}{-13}~~ = ~~\stackrel{\stackrel{m}{\downarrow }}{-\cfrac{3}{13}}\implies y+9=\cfrac{3(-13)}{-13} \\\\\\ y+9=3\implies {\LARGE \begin{array}{llll} y = -6 \end{array}}`