Question

What is the domain of f(x) = 7/(x-10) - 7

Answer 1

`{ \qquad\qquad\huge\underline{{\sf Answer}}}`

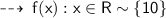
Here we go ~

For the given function :

`\qquad \sf  \dashrightarrow \: f(x) = (7)/(x - 10) - 7`

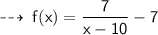
f(x) is defined for all real values of x, except the value of x for which the denominator becomes 0, because if denominator is 0, the function at that instant will be undefined.

that is : f(x) is not defined when :

`\qquad \sf  \dashrightarrow \: x - 10 = 0`

`\qquad \sf  \dashrightarrow \: x = 10`

Hence, f (x) is defined for all real values of x except 10

It can be represented as :

`\qquad \sf  \dashrightarrow \: f(x) : x \in R \sim \{10 \}`