Answer:
The slope of line that passes through the points (2, -4) and (5, 2) is 2.
Step-by-step explanation:
Here's the required formula to find slope line :
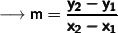
As per given question we have provided that :
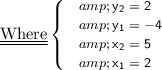
Substituting the values in the formula to find slope line that passes through the points :
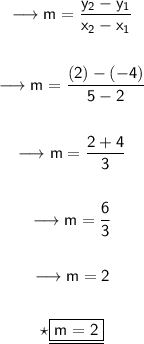
Hence, the slope of line that passes through the points (2, -4) and (5, 2) is 2.
