Question

Find the instantaneous rate of change of the function

f(x)=2x^3−3x at the point (2,10)

A. -3

B. 5

C. 9

D. 21

E. 33

Answer 1

We can find the instantaneous rate of change at
`x=2` by evaluating the limit

`\displaystyle \lim_(x\to2) (f(x) - f(2))/(x - 2)`

(i.e. the definition of the derivative)

Since

`f(x) = 2x^3 - 3x \implies f(2) = 10`

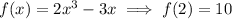
we have

`\displaystyle \lim_(x\to2) (f(x) - f(2))/(x - 2) = \lim_(x\to3) (2x^3 - 3x - 10)/(x - 2)`

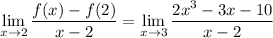
Note that
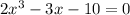`2x^3-3x-10=0` when
`x=2`, which means
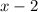`x-2` divides the numerator exactly. By polynomial division, we can show

`(2x^3 - 3x - 10)/(x - 2) = 2x^2 + 4x + 5`

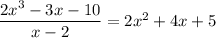
Then in the limit, we can write

`\displaystyle \lim_(x\to2) (f(x) - f(2))/(x - 2) = \lim_(x\to2) ((x-2) (2x^2 + 4x + 5))/(x - 2)`

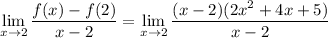
As
`x\\eq2`, we can cancel the factors of
`x-2`.

`\displaystyle \lim_(x\to2) (f(x) - f(2))/(x - 2) = \lim_(x\to2) (2x^2 + 4x + 5)`

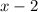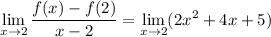
The function in the remaining limit is continuous at
`x=2`, so we can directly substitute
`x=2` to get its value,

`\displaystyle \lim_(x\to2) (f(x) - f(2))/(x - 2) = 2\cdot2^2 + 4\cdot2 + 5 = \boxed{21}`

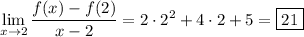
(D)