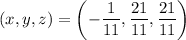Answer:
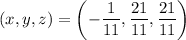
Explanation:
Given:
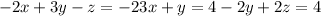
Therefore:
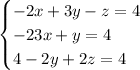
Rewrite Equation 2 to make x the subject:
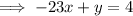
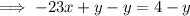
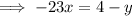
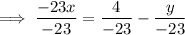
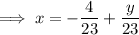
Rewrite Equation 3 to make z the subject:

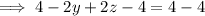
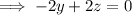

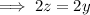
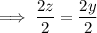
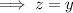
Substitute the found expressions for y and z into Equation 1 and solve for y:
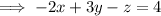
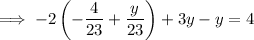

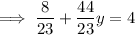
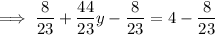
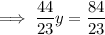
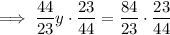

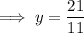
Therefore, as y = z:
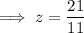
Substitute the found value of y into the found expression for x and solve for x:
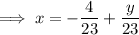
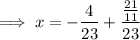
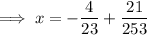
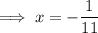
Therefore, the final solution is: