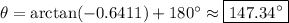Question

Please Help acellus is torture.

Answer 1

The angle made by the vector is
`\theta` such that

`\tan(\theta) = (18.4\,\rm m)/(-28.7\,\rm m) \approx -0.6411`

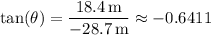
Before taking the inverse tangent of both sides, recall that
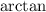`\arctan` returns a number between -π/2 and π/2 radians, or -90° and +90°. The vector in the diagram clearly makes an angle between 90° and 180°, however, so we use the fact that tangent has a period of π rad / 180° to write

`\theta \approx \arctan(-0.6411) + 180^\circ n`

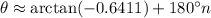
where
`n\in\Bbb Z`.

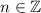Now,

`\arctan(-0.6411) \approx -0.5701 \,\mathrm{rad} \approx -32.6639^\circ`

Add 180° to this to recover the correct angle.

`\theta = \arctan(-0.6411) + 180^\circ \approx \boxed{147.34^\circ}`