Answer:
(a) Choice C is correct
(b) f(x) = -8 Point is (1, -8)
(c) x =11 Point is at (11,2)
(d) Domain of f is x is
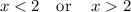
In interval notation
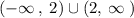
(e) x intercept is at x = -7
(f) y-intercept is at
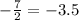
Explanation:
(b) Plug in x = 1 into the equation
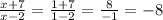
(c) If f(x) = 2 then we get
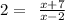
==> 2(x-2) = x + 7
==> 2x - 4 = x + 7
==> 2x - x = 7 +4 =11
==> x = 11
(d) Domain of f is all values of x -∞ < x < ∞ except at x = 2 since at x = 2 the denominator is 0 and division by zero is undefined; So we can say the domain is x < 2 or x >2
(e) To get the x-intercept set y = 0 and solve for x
We get (x+7)/(x-2) = 0
x+7 = (x-2)(0) = 0
x = -7 and the x intercept is at point (-7, 0)
(f)
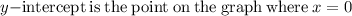
Substitute for x = 0 to get y = (0+7)/(0-2) = -7/2