Question

14x-3y=-2
4x + 5y = 14
solve each system of equations by elimination

Answer 1

x =
`(16)/(41)`

y =
`(102)/(41)`

Explanation:

To use elimination I need either the x terms to be inverses of each other or the y terms to be inverses of each other. There are a number of ways to do this. I am going to focus on the y's. If I multiple the first equation through by 5 and the second equation through by 3. I will make equivalent equations that will allow the y terms to be eliminated.

14x - 3y = -2

5(14x - 3y) =5(-2)

70x - 15y = -10

4x + 5y = 14

3(4x + 5y) = 3(14)

12x +15y =42

Add together the 2 bold equations above

70x - 15y = -10

12x + 15y = 42

82x = 32 Divide both sides by 82

x =
`(32)/(82)` =
`(16)/(41)`

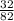Plug this in either equation to solve for y

y =
`(102)/(41)`