Answer:

Explanation:
Firstly finding the side lengths of the square by Pythagoras Theorem since the base and perpendicular of the triangle is given and hypotenuse is to be found which is also the side length of the square.
Pythagoras Theorem is:
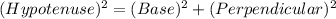
Where, base = 2, perpendicular = 3
(H)² = (2)² + (3)²
(H)² = 4 + 9
(H)² = 13
Taking sqrt on both sides
H = 3.6
All the sides have to be 3.6 since its a square.
Now,
Area of the square:
= Length × length
= 3.6 × 3.6
= 12.9
≈ 13
![\rule[225]{225}{2}](https://img.qammunity.org/2023/formulas/english/college/eq413d752mwtrwwenrzwldxt4w1olmf1b3.png)
Hope this helped!
~AH1807