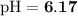Question

. Calculate the pH value of a solution in which [OH- ] = 1.49 × 10−8M

Answer 1

The pH of the solution is 6.17.

Step-by-step explanation:

To find the pH of the solution, we have to first calculate its pOH using the formula:

`\boxed{\mathrm{pOH = - log_(10)[OH^-]}}`

Substituting the given value of [OH⁻] into the formula, we get:

`\mathrm{pOH = -log_(10)(1.49 * 10^(-8))}`

`= \bf 7.83`

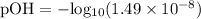
Now that we have the value of the pOH of the solution, we can find its pH using the following formula:

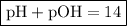
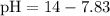
`\boxed{\mathrm{pH + pOH = 14}}`

∴
`\mathrm{pH} + 7.83 = 14`

⇒
`\mathrm{pH } = 14 - 7.83`

⇒
`\mathrm{pH } = \bf 6.17`