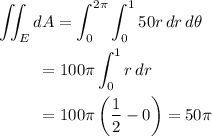Solve for
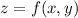 in the plane equation.
in the plane equation.
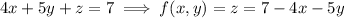
Let
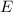 be the set of points in the plane
be the set of points in the plane
 bounded by the ellipse
bounded by the ellipse
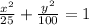 , i.e.
, i.e.
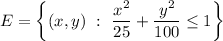
Then the area of the plane bounded by the elliptic cylinder is
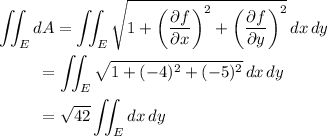
which is simply √42 times the area of the ellipse in the plane
 . This ellipse has a minor axis of length 5 and a major axis of length 10, so its area is π•5•10 = 50π, and so the area of the plane in question is 50√42 π.
. This ellipse has a minor axis of length 5 and a major axis of length 10, so its area is π•5•10 = 50π, and so the area of the plane in question is 50√42 π.
To confirm this result: In polar coordinates, with

the area element is
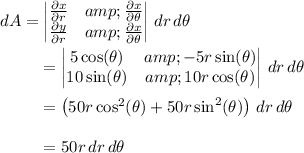
The ellipse can be parameterized by
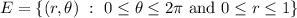
so that the integral for the area of the ellipse in the plane
 is
is