1 Answer

Sander Vanhove · Answer 1 · 2023-04-29T08:04:47+0000

Answer:

Solution: x = 9 Extraneous solution: x = 1

Explanation:

Given equation:

√(4x)=x-3

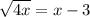
Square both sides:

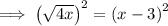
$\implies \left(√(4x)\right)^2=\left(x-3\right)^2$

$\implies 4x=x^2-6x+9$

Subtract 4x from both sides:

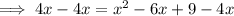
$\implies 4x-4x=x^2-6x+9-4x$

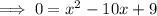
$\implies 0=x^2-10x+9$

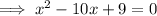
$\implies x^2-10x+9=0$

Split the term in x:

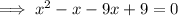
$\implies x^2-x-9x+9=0$

Factor the first two terms and the last two terms separately:

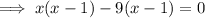
$\implies x(x-1)-9(x-1)=0$

Factor out the common term (x - 1):

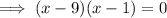
$\implies (x-9)(x-1)=0$

Apply the zero-product property:

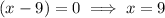
$(x-9)=0 \implies x = 9$

$(x-1)=0 \implies x=1$

Substitute the found values of x into the original equation to verify the solutions:

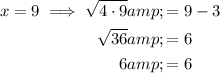
$\begin{aligned}x=9 \implies √(4 \cdot 9) & = 9-3\\√(36) & = 6\\6 & = 6\end{aligned}$

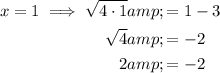
$\begin{aligned}x=1 \implies √(4 \cdot 1) & = 1-3\\√(4) & = -2\\ 2 & = -2\end{aligned}$

Therefore, the only valid solution is x = 9:

Extraneous solution: A solution from the process of solving the equation that is not a valid solution to the original equation.

Please log in or register to add a comment.